
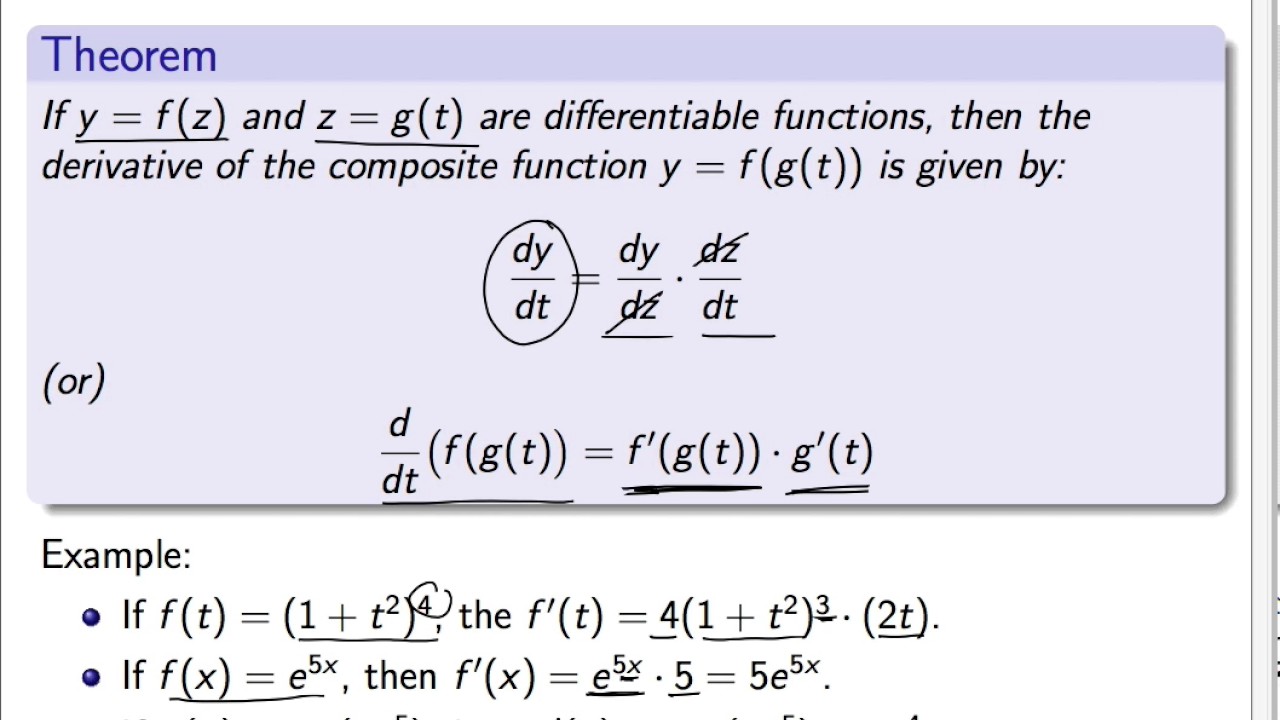
Yes, my understanding is that the definition of infinitesimals in non-standard analysis gives rise to different orders of infinitesimals.Ĥ. Are there also multiple orders of infinitesimals? Can one infinitesimal value be smaller than another? It is generally agreed that there are multiple orders of infinity. However, non-standard analysis is an alternative formulation of calculus that gives a rigorous definition of infinitesimals and uses this as the foundation.ģ. Infinitesimals do not exist in the system of real numbers. Not in the standard formulation of calculus. Does this mean, though, that the tangent line to a curve at a particular point is actually a line defined by two points separated by an infinitesimal distance?
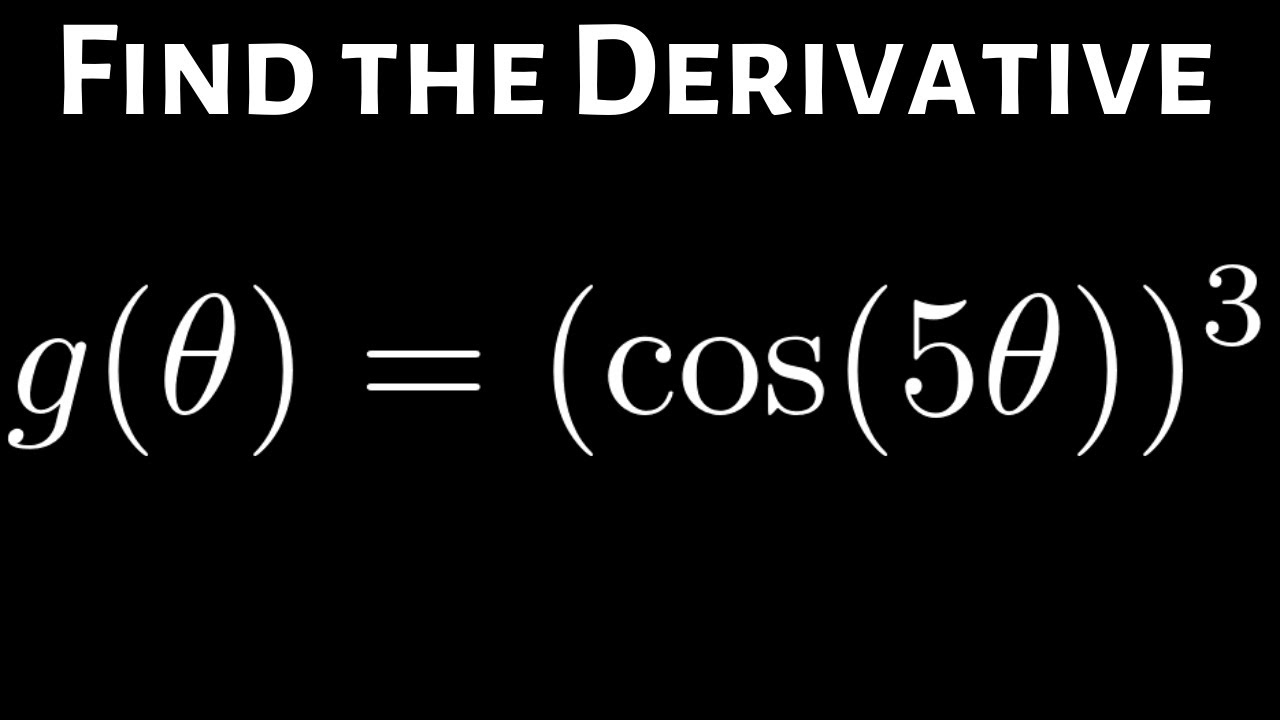
Is there a generally agreed upon, well-defined concept of infinitesimal values in mathematics? As I understand it, in differential calculus, we use the concept of the limit 1/n as n goes to infinity to define the concept of a derivative. I hope some of these questions made sense, and maybe you could point me to some relevant information on this topic because the Wikipedia entry on infinitesimals is rather poor.ġ. If points of an infinitesimal size exist, could the length of a line be defined as sum of the length of an infinite number of infinitesimal points? I imagine this would cause some difficulty, as the number of points between 0 and 1 are mappable to the number of points between 0 and 2, though clearly the lines are not the same length. If infinitesimal quantities exist, would it be reasonable to posit a point which has an infinitesimal length in every dimension? Similarly, could a one-dimensional horizontal line be seen to have an infinitesimal height (as well as width and metrics in every other dimension)? If not, is the line's height in other dimensions 0? Is there a difference between having a height of 0 and an infinitesimal height? Most mathematical definitions that I've seen for a "point" assume that a point has no size in any dimension. They draw upon widely different sections of mathematics.I've recently been wondering about infinitesimal quantities, and there are a few things that I'm having difficulty wrapping my head around. And in addition to this there are such new emerging subdisciplines as "experimental mathematics", "CFD", "completely integrable systems", "chaos, synergetics and large-scale order", which are almost impossible to fit into the existing classification schemes. Further, the kind and level of sophistication of mathematics applied in various sciences has changed drastically in recent years: measure theory is used (non-trivially) in regional and theoretical economics algebraic geometry interacts with physics the Minkowsky lemma, coding theory and the structure of water meet one another in packing and covering theory quantum fields, crystal defects and mathematical programming profit from homotopy theory Lie algebras are relevant to filtering and prediction and electrical engineering can use Stein spaces. It also happens, quite often in fact, that branches which were thought to be completely disparate are suddenly seen to be related. However, the "tree" of knowledge of mathematics and related fields does not grow only by putting forth new branches. Growing specialization and diversification have brought a host of monographs and textbooks on increasingly specialized topics. The Scandal of Father 'The Hermit Clad in Crane Feathers' in R. perhaps you will find the final question. Then one day, that they can't see the problem. Approach your problems from the right end It isn't that they can't see the solution.


 0 kommentar(er)
0 kommentar(er)
